Gabriel's Horn is a paradoxical mathematical object. That's because it has a finite volume and an infinite surfacearea.

In other words: if Gabriel's Horn is a paint pot, more paint is used to paint its surface than to fill it up! Or, to repeat myself, Gabriel's Horn can never contain enough paint to paint its own surface - no matter how thin a layer of paint is used!

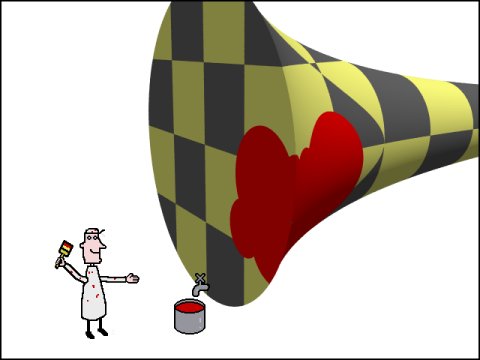
Gabriel's Horn is constructed by taking the graph for y=(1/x), and throw everything away where x<1, and then rotate the rest around the x-axis.

The volume can be calculated to be

V = π ≈ 3.1415

and, as mentioned, the surfacearea to be

A = ∞

---

By this I have said what I got to say, but those who want to see mathematical proof can read on...

A curve rotated about the x-axis has

volume: V = ∫

b a

π·(r x)2 dx

area:   A = ∫

b a

2π·(r x)·√1 + (r' x)2 dx

For Gabriels Horn: r x = 1/x , a = 1 and b > 1

V = ∫

b 1

π·(1/x)2 dx

= π·[-1/x]

b 1

  = π·( -1/b + 1 )
so V → π when b → ∞

A = disk + tube

= π·(1/1)2 + ∫

b 1

2π·(1/x)·√1 + (-1/x2)2 dx

> π + ∫

b 1

2π·(1/x) dx

= π + 2π·[ln x]

b 1

  = π + 2π·(ln b)
so A → ∞ when b → ∞

QED